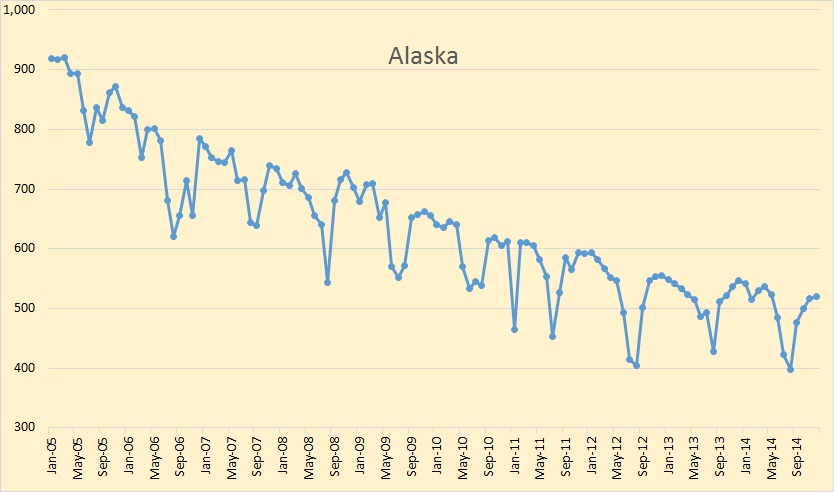
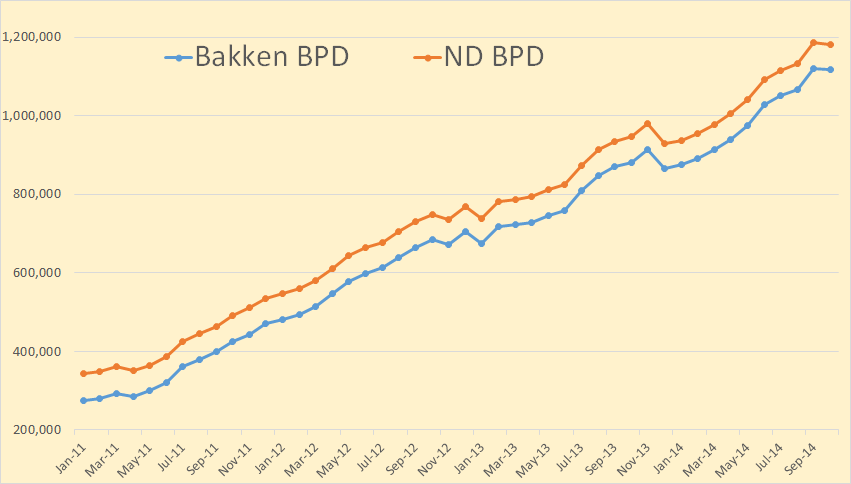
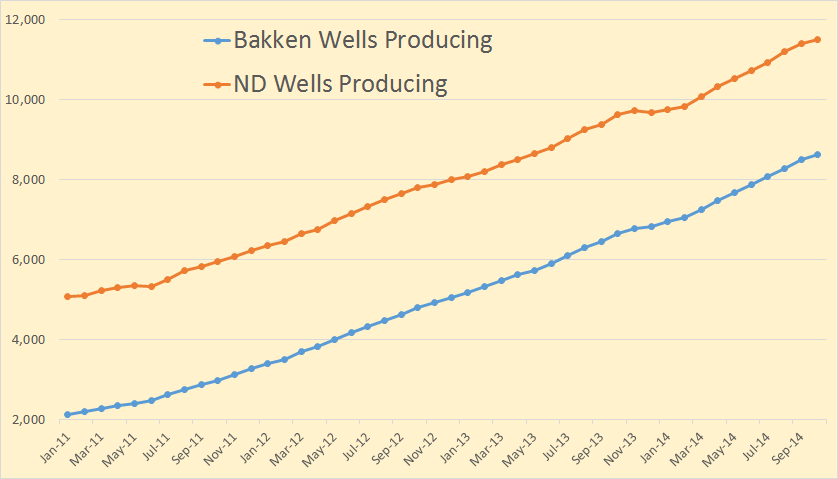
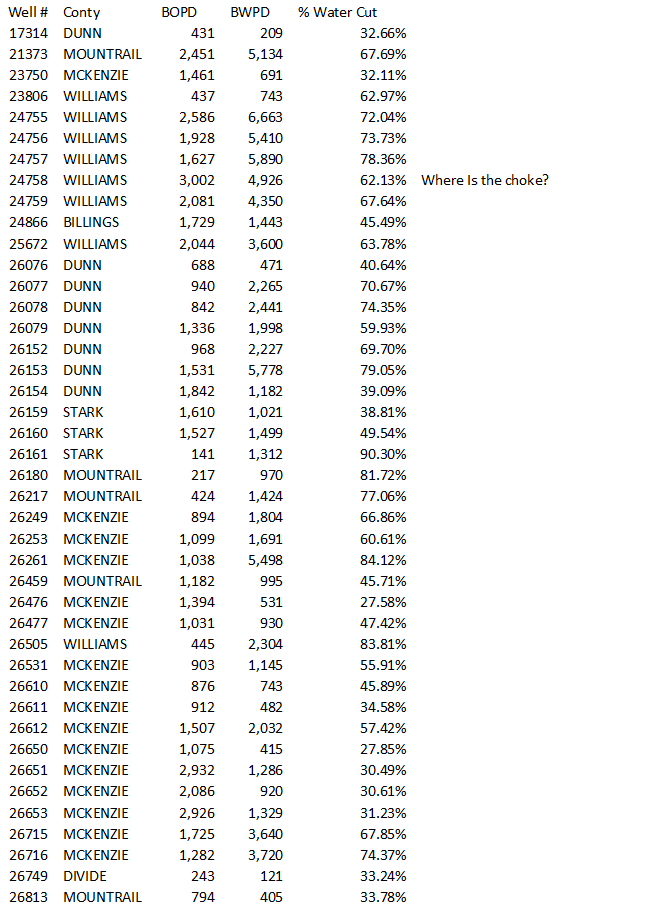
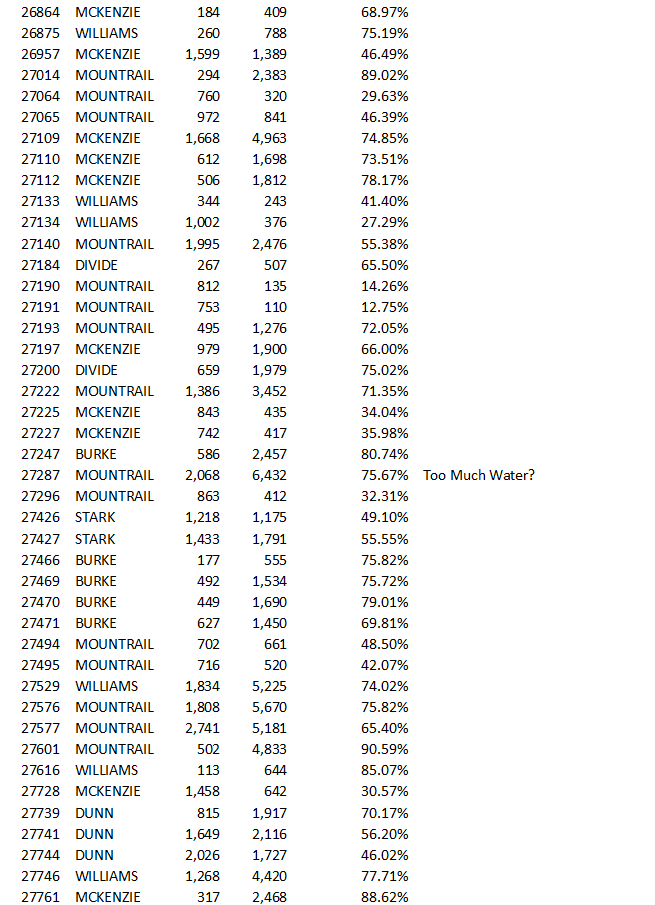
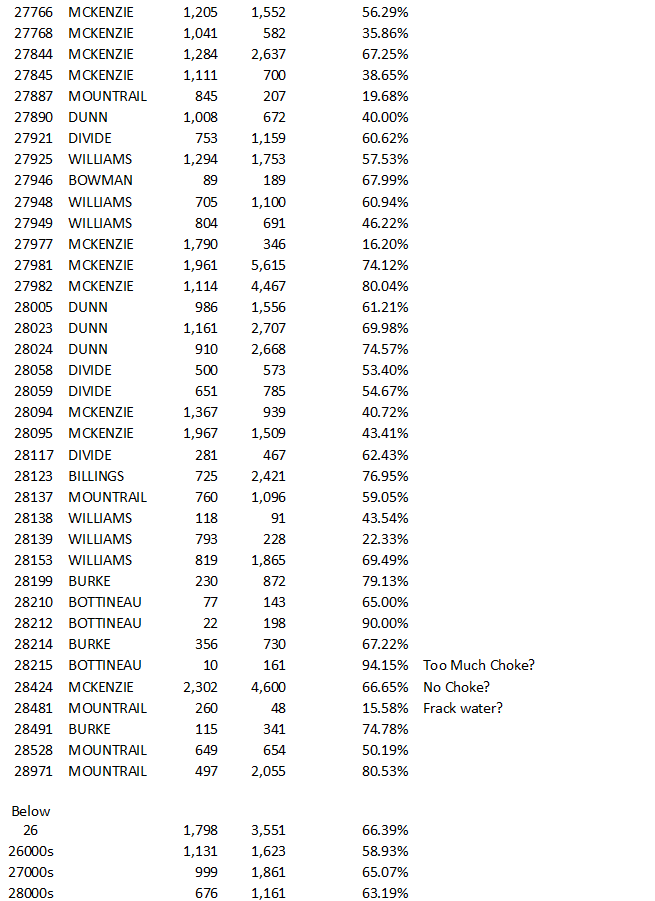
The EIA came out with its final update of Annual Energy Outlook 2015. It seems that the EIA is extremely optimistic concerning future US crude oil production.
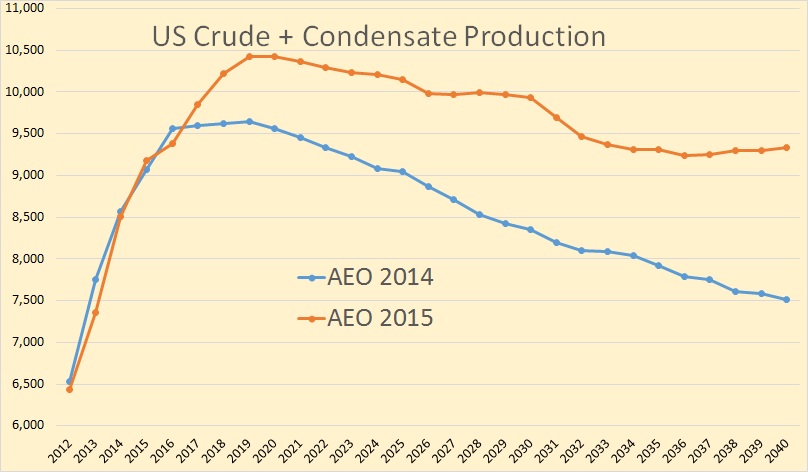
Here is a comparison with AEO 2014. The EIA still expects US crude production to peak in 2019 but at 10,472,000 bpd or 824,000 barrels per day higher than the expected last year. But the biggest difference is in the EIA’s change in decline expectations. They now expect the US to be producing 9,329,000 bpd in 2040 or 1,812 higher than they had 2040 production last year. This is the EIA’s reference, or most likely case.
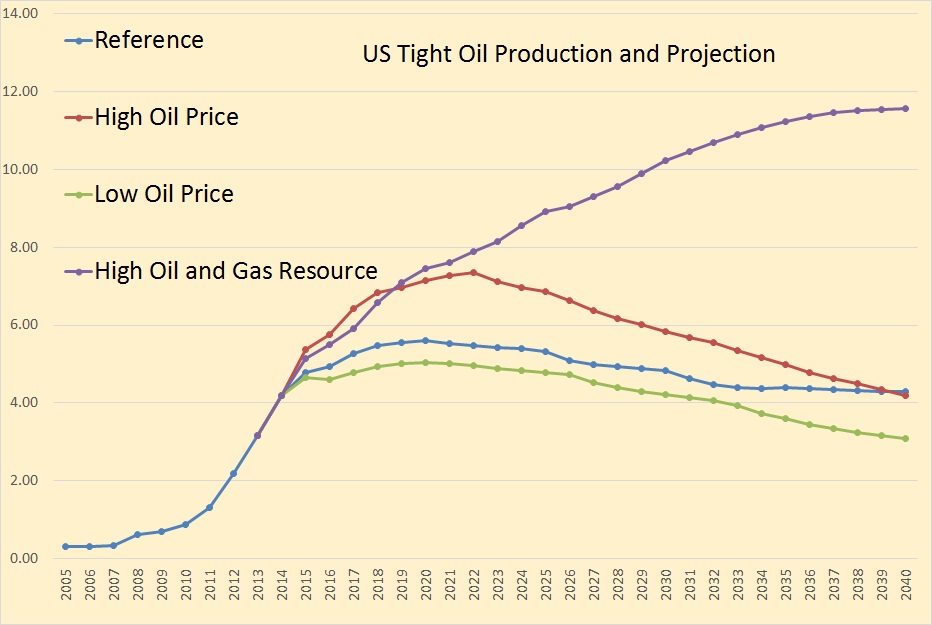
Production from tight formations leads the growth in U.S. crude oil production across all AEO2015 cases. The path of projected crude oil production varies significantly across the cases, with total U.S. crude oil production reaching high points of 10.6 million barrels per day (bbl/d) in the Reference case (in 2020), 13.0 million bbl/d in the High Oil Price case (in 2026), 16.6 million bbl/d in the High Oil and Gas Resource case (in 2039), and 10.0 million bbl/d in the Low Oil Price case (in 2020).